-
28 Jun 2021 - 1 Jul 2021
8:00 am - 5:00 pm
Organisers:
Dan Betea (KU Leuven)
Greta Panova (University of Southern California)
Leonid Petrov (University of Virginia)
Tomohiro Sasamoto (Tokyo Institute of Technology)
Michael Wheeler (University of Melbourne)
Program Description:
The overall goal of the program is to advance the methods of integrability and combinatorics towards structural and asymptotic results across a wide range of finite temperature models of statistical physics. Here the notion of “finite temperature” might have different meanings in different situations, and a complete understanding of the range of finite temperature models and related asymptotic phenomena is a work in progress. The potential impact of the program is based on previous very successful treatment in the past 20 years of “infinite temperature” models (based on, e.g., sums of independent random variables) in classical and modern probability theory, and of “zero temperature” models (e.g. last passage percolation). The latter were analyzed using the methods of integrability and combinatorics, and currently these methods are being extended by a number of groups towards more complicated “finite temperature” settings, in order to capture new universal phenomena. Our aim is to bring together members of these groups, and produce further advances in this direction. It is expected that new finite-temperature universal phenomena will apply to a wide range of real-world settings, such as to the structure of ice and other condensed matter models, magnetism, quantum spin systems, thermodynamics, and polymers.
Program Structure:
The conference will consist of a series of mini-courses (with exercise sessions) given by:
Vadim Gorin (University of Wisonsin at Madison, USA)
Title: General beta random matrix theory
Abstract: In random matrix theory the role of the inverse temperature is played by the parameter beta, which takes values 1/2/4 when we deal with real/complex/quaternionic matrices. In this course we explain how one can take beta to be an arbitrary positive real and what does it mean to add, multiply, or cut corners from general beta random matrices. Both low temperature and high temperature asymptotics will be discussed and will lead to intriguing limiting objects.
Jérémie Bouttier and Grégory Schehr (CEA and ENS de Lyon, and University of Paris-Saclay, France)
Title: Some aspects of noninteracting trapped fermions at finite temperature
Abstract: Noninteracting fermions in a 1D confining potential form arguably one of the simplest models in many-body quantum statistical physics. In this mini-course, we will review some of the methods used to study them, with an emphasis on their edge fluctuations at finite temperature.
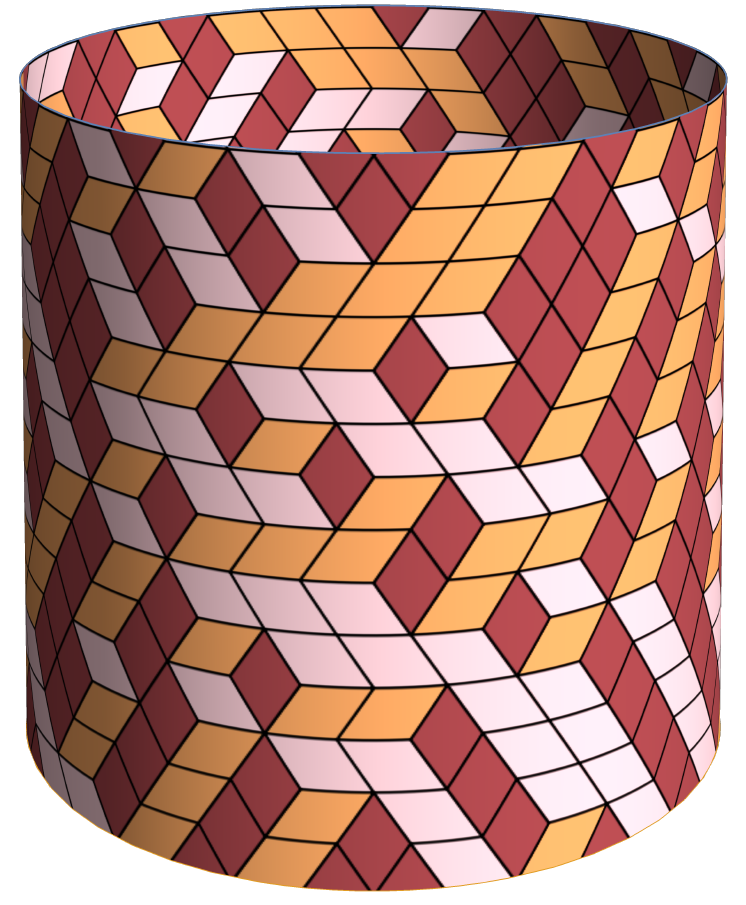
Michael Wheeler (University of Melbourne, Australia)
Title: Integrable vertex models on the cylinder
Abstract: Stochastic vertex models in the quadrant have been very topical in integrable probability in the last decade, with a host of reductions to one-dimensional particle processes. In this course we examine the combinatorial implications of placing these models on a cylinder. Connections to (non)symmetric Macdonald theory and LLT polynomials will be discussed, as well as the difficult problem of expanding one family of symmetric functions in terms of another.
Evgeni Dimitrov (Columbia University)
Alexandr Garbali (University of Melbourne)
Andrew Gitlin (University of California at Berkeley)
Alisa Knizel (University of Chicago)
Alexandre Krajenbrink (SISSA)
William Mead (University of Melbourne)
Alejandro Morales (University of Massachusetts Amherst)
Matteo Mucciconi (Tokyo Institute of Technology)
GaYee Park (University of Massachusetts Amherst)
Marianna Russkikh (MIT)
Travis Scrimshaw (University of Queensland)
Sofia Tarricone (Université d’Angers and Concordia University)
Harriet Walsh (ENS de Lyon)
Nikos Zygouras (University of Warwick)
Times: all times are in UTC
Zoom links: Communicated to registered participants in advance via email
In Person Participant list:
Jan de Gier (The University of Melbourne)
Alexandr Garbali (The University of Melbourne)
Will Mead (The University of Melbourne)
Weiying Guo (The University of Melbourne)
Paul Zinn-Justin (The University of Melbourne)
Ajeeth Gunna (The University of Melbourne)
Jules Lamers (The University of Melbourne)
Peter McNamara (The University of Melbourne)
Johanna Knapp (The University of Melbourne)
ASSOCIATED EVENTS
MATRIX Wine and Cheese Afternoon 15 June 2021
On the first Tuesday of each program, MATRIX provides a pre-dinner wine and cheese afternoon. Produce is locally-sourced to showcase delicacies from the region.